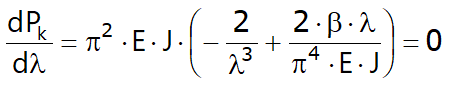Due to their decided slenderness, it is opportune to verify the stability of elastic equilibrium of Tubifix minipiles embedded in the terrain.In the interests of safety, computation assumes that the trunk head be hinged/pinned into the foundation while the bulb be embedded in an elastic medium. Critical load is then determined by the following relationship:
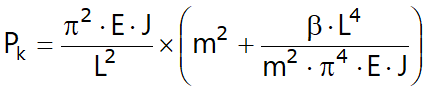
where:
Pk = Critical load
E = Steel elastic modulus
J = Moment of inertia of the reacting section
L = Length between the extremities of the minipile that are assumed to be bound
b = Terrain reaction modulus per unit of lateral displacement
m = Integer number of halfwaves of trunk flexion
![]()
Dp= Diametro di perforazione
K= Modulo di Winkler
When L reaches very high values, the assumption of single deformation (m=1) brings Pk to unlikely values. The minimum value of Pk is obtained for m >1.
By intoducing the dimension l = L / m (halfwave length):
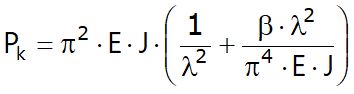
To obtain the value of Pk from the above lambda (l) may be considered as a continuous variable in whose relation Pk may be derived:
|
|
|
|
Di = Internal tube diameter
De = External tube diameter
Dp = Drill dimeter
n = Homogeneity modulus steel concrete
Ki = Coefficient between 0 and 1 that indcates the degree of steel concrete participation.
© GeoStru